Many plants show the Fibonacci numbers in the arrangement of the leaves around the stem. Some pine cones and fir cones also show the numbers, as do daisies and sunflowers. Some coniferous trees show these numbers in the bumps on their trunks. Conceptually, that is not unlike our findings in this experiment. Further, during the testing phase, this study found this technique consistently caused enough dermo-epidermal shear to cause displacement of the fatty dermis and the creation of whorl patterns.
The fascination with the golden spiral symbol has led many artists to use it in their masterpieces. There’s a good chance that you’ve already seen the symbol as overlays on various art forms, from the Parthenon to the Mona Lisa. Unfortunately, there are many confusing claims about the subject, so we’ll help you decide whether they’re grounded in myth or math. We touched on the 2 types of spirals i suggest looking for in nature, and we can also look at the geometry of different spirals and how they relate to measuring and form. There are Archimedean spirals, logarithmic or Fibonacci and we touched a little on the Golden spiral. There are simple drawing exercises for students in a walorf inspired learning environment who can create spirals with simple tools and the Waldorf curriculum encourages the Advent spiral in its annual celebrations.
For that reason, fractals are often likened to Russian nesting dolls. Look at the array of seeds in the center of a sunflower and you’ll notice they look like a golden spiral pattern. Amazingly, if you count these spirals, your total will be a Fibonacci number. Divide the spirals into those pointed left and right and you’ll get two consecutive Fibonacci numbers.
While others believe that it is just a very strange coincidence, many scientists and researchers regard it as an evidence of a Master Mathematician or a Creator. After all, the intelligent design in nature is complex, and it might seem illogical to some to think that it came about by chance. With some of these flatter bivalve shells the actual spiral is difficult to see because it is so distorted from what we expect a spiral to be. Yet spirals come in all forms, it takes just three factors to change the shape of a spiral.
Human Hand
Mozart divided a striking number of his sonatas into two parts whose lengths reflect the golden ratio, though there is much debate about whether he was conscious of this. In more modern times, Hungarian composer Bela Bartok and French architect Le Corbusier purposefully incorporated the golden ratio into their work. Romanesque Brocolli/Cauliflower (or Romanesco) looks and tastes like a cross between brocolli and cauliflower. Each floret is peaked and is an identical but smaller version of the whole thing and this makes the spirals easy to see. In mathematics and the arts, two quantities are in the golden ratio if the ratio between the sum of those quantities and the larger one is the same as the ratio between the larger one and the smaller. This kind of fractal design, like the spiraling of succulents, helps trees optimize their exposure to sunshine and prevents top branches from shading out lower ones.

Dr. Stephen Marquardt, a former plastic surgeon, has used the golden section, that enigmatic number that has long stood for beauty, and some of its relatives to make a mask that he claims is the most beautiful shape a human face can have. These numbers are precisely those of the Fibonacci sequence (the bigger the numbers, the better the approximation) and the choice of the fraction depends on the time laps between the appearance of each of the seeds at the center of the flower. Trees are, in fact, fractal from their seeds to their roots to their leaves to their canopy. If you look closely at the veins of the leaves, you’ll notice just how self-similar they are. The tiniest ones look like the main midrib (the midline vein), and the midrib looks like the tree trunk with its branches. The curves, experts have found, are always six times the width of the channel.
A list of Spirals in Nature
Interestingly, when we had traced out all the patterns and then superimposed all our tracings, one on top of each other to create a composite image, we noted a distinctive logarithmic spiral pattern. The results are presented here—the composite image created by overlaying all our tracings show a clear logarithmic spiral pattern, in our case very close to a golden spiral pattern seen in nature. “Fig 2” shows each individual tracing, followed by a composite image, finally overlaid by a golden spiral. Each tracing, when analyzed retrospectively did indeed conform to a portion of a golden spiral. The mechanical theory of scalp expansion causing whorls, while hypothesized, has never been re-created, to our knowledge, in a controlled experiment on skin.
Every human has two hands, each one of these has five fingers, each finger has three parts which are separated by two knuckles. Outside of nature, you’ll see these fractal designs in a soapy bath or while doing dishes in the sink. In nature, bubbles that occur when ocean waves break or where raindrops have fallen create a self-similar pattern with thin films of liquid separating various-sized gas pockets. Large bubbles are interspersed with small bubbles and those small bubbles interspersed with even smaller bubbles, and so forth. Fractals are known as geometric shapes that display similarity through the full range of scale—that is, they look the same no matter how big or how small they are. The further along the Fibonacci sequence you go, the closer the ratio between successive numbers in the sequence gets to Phi, or 1.618, which is the Golden Ratio.
Snail shells
Also, the structure is in ruins, which makes its exact dimensions subject to some estimation. A logarithmic spiral, equiangular spiral, or growth spiral is a self-similar spiral curve that often appears in nature. But, don’t let the maths and numbers put you off, what is important is to look and to be amazed by these spirals in nature, then your wonder will surely grow. You will see how the patterns of spirals in nature indicate the mathematical rules of growth. Despite these numerous appearances in works of art throughout the ages, there is an ongoing debate among psychologists about whether people really do perceive the golden shapes, particularly the golden rectangle, as more beautiful than other shapes.
You have one nose, two eyes, three segments to each limb and five fingers on each hand. The proportions and measurements of the human body can also be divided up in terms of the golden ratio. DNA molecules follow this sequence, measuring 34 angstroms long and 21 angstroms wide for each full cycle of the double helix. From Canada, Ty was born in Vancouver, British Columbia in 1993. From his chaotic workspace he draws in several different illustrative styles with thick outlines, bold colours and quirky-child like drawings. Ty distils the world around him into its basic geometry, prompting us to look at the mundane in a different way.
Related Articles
Another approximation is a Fibonacci spiral, which is constructed slightly differently. A Fibonacci spiral starts with a rectangle partitioned into 2 squares. In each step, a square the length of the rectangle’s longest side is added to the rectangle.
The phenomenon is expertly demonstrated by copper crystals, which branch out in all directions like tree limbs. Each “twig” is a new growth point—as it branches out, it develops into solid metallic copper. Because of their arborescent nature and unique reddish-brown color, copper crystals are often grown for art. The Fibonacci sequence works in nature, too, as a corresponding ratio that reflects various patterns in nature — think the nearly perfect spiral of a nautilus shell and the intimidating swirl of a hurricane. Experts also say that a galaxy or occasional storm cloud that fits a part of a golden spiral shouldn’t be a conclusion that all galaxies and hurricanes are based on the golden ratio. The golden spiral is unique in its mathematical properties and proves that we live in a universe governed by mathematical laws.
Regardless of the science, the golden ratio retains a mystique, partly because excellent approximations of it turn up in many unexpected places in nature. The spiral inside a nautilus shell is remarkably close to the golden section, and the ratio of the lengths of the thorax and abdomen in most bees is nearly the golden ratio. Even golden spiral in nature a cross section of the most common form of human DNA fits nicely into a golden decagon. The golden ratio and its relatives also appear in many unexpected contexts in mathematics, and they continue to spark interest in the mathematical community. Plants do not know about this sequence – they just grow in the most efficient ways.
- In keeping with such genetic theories, the number of whorls on a horse’s face and the direction of such patterns are used to gauge equine temperament such as calmness, enthusiasm or wariness [11].
- The tiniest ones look like the main midrib (the midline vein), and the midrib looks like the tree trunk with its branches.
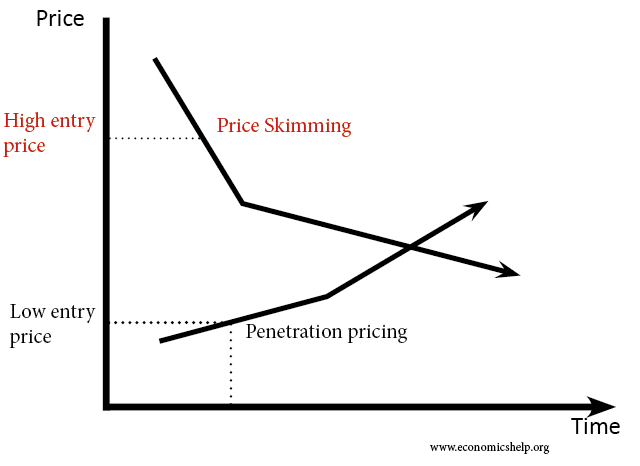
- Specifically, a golden spiral gets wider (or further from its origin) by a factor of j for every quarter turn it makes.
- You have no doubt noticed the satisfying spiral of their scales, which reflect the seeds they protect.
- In the mid-00s, a mathematician hypothesized that the spiral pattern of both plants and fingerprints occurred for the same reason, which was to relieve stress.
The more we understand the math behind the symbol, the more we’ll appreciate its appearances in nature and arts. In geometry, a golden spiral is a logarithmic spiral whose growth factor is φ, the golden ratio.[1] That is, a golden spiral gets wider (or further from its origin) by a factor of φ for every quarter turn it makes. The golden spiral plays a role in the geometry of fractals, a complex pattern that repeats forever. American mathematician Edmund Harriss became popular for his fractal curve based on the golden spiral, now known as the Harriss Spiral. It’s said that he aimed to draw branching spirals that look aesthetically appealing, but he ended up with a unique spiral by using a mathematical process. In 1800s, German mathematician Martin Ohm called the special number 1.618 golden, likely because it has always existed in mathematics.
But this ratio is derived from the Fibonacci sequence named after the scientist to discover both the things. Moreover, Phyllotaxis was connected with the golden spiral because it includes the consecutive leaves or leaflets which are being distinct by golden angle. Golden spiral ratio shows a sense of beauty and some real masterpieces of art. The explanation is linked to another famous number, the golden mean, itself intimately linked to the spiral form of certain types of shell. Mathematician George Markowsky pointed out that both the Parthenon and the Great Pyramid have parts that don’t conform to the golden ratio, something left out by people determined to prove that Fibonacci numbers exist in everything.

Recent Comments